Algebra Mathematics
More Common Polynomial Products
Square of a Trinomial
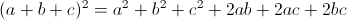
Cube of a Binomial

Difference of Cubes
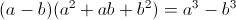
Sum of Cubes
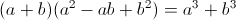
Here, we have four more commonly used polynomial product formulas from algebra that generalize the prior formulas for common products. The first formula is the square of a trinomial and is similar to the square of a binomial formula. The second formula is the cube of a binomial. It is like the square of a binomial, but to a higher exponent. The third formula is the difference of cubes, and it is simlar to the difference of squares formula. The fourth formula is the sum of cubes, and it can be derived directly the difference of cubes formula. All of the formulas are special cases of more general formulas that will be derived later.
Derivations
Square of a Trinomial
For the first equality, we use the additive associative proerty to group (b + c) together. Then we apply the square of a binomial formula with the terms a and (b + c) to get the second equality. For the third equality, we apply the distributive property to turn 2a(b + c) into 2ab + 2ac. Then another application of the square of a binomial formula on the last term gives us the fourth equality. The fifth and final equality comes from rearranging the terms through additive commutativity.
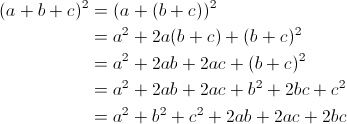
Cube of a Binomial
Starting with the binomial cubed, we can break off one of the (a + b) terms by using the definition of exponents or the properties of exponents to get the first equality. For the second equality, we apply the square of a binomial on (a + b) squared. Distribute the (a + b) for the third equality. Again, use the distributive property several times and use multiplicative commutativity to get the fourth equality. The fifth equality follows after some rearrangement using the additive commutativity property. Additive associativity allows us to group the terms for the sixth equality. Distributivity gives us the seventh equality. The eight and final equality follows from the fact that (2 + 1) = 3 and (1 + 2) = 3.
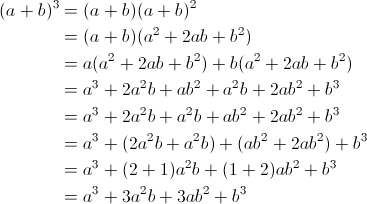
Difference of Cubes
For the first equality, we apply the distributive property. Then a few more applications of distributivity give us the second equality, along with the multiplicative commutativity property and product of an additive inverse. The third equality follows from additive associativity and additive inverse of a sum. We can eliminate the middle terms because they are additive inverse to get the fourth equality. The fifth and final equality follows by the additive identity property.
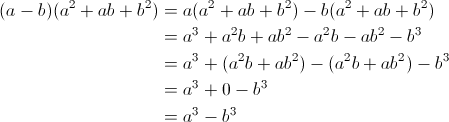
Sum of Cubes
To get the first equality, we use the additive inverse of an additive inverse twice. The additive inverse of a product gives us the second equality. The third equality follows from the a change to subtraction notation and two applications of the additive inverse of a product. Using a and -b in the difference of cubes formula gives us the fourth equality. Finally, the fifth and final equality follows from several applicaitons of both the additive inverse of an additive inverse and the additive inverse of a product.
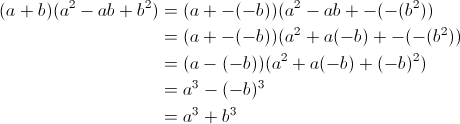
© 2007–2024 XoaX.net LLC. All rights reserved.
